Primary Mathematics

The Concrete – Pictorial – Abstract Approach:

Students engage with mathematical concepts by first handling physical objects, then representing mathematical ideas using diagrams, and finally using abstract representations. Through the use of concrete materials and visual representations, students are able to see and make sense of the math and the abstract representations.

Visual Models including Bar Models

Visual models such as number bonds, bar models and fraction models are hallmarks of Primary Mathematics. These models help students visualize and understand abstract math concepts.

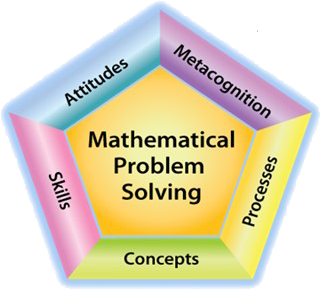
Problem Solving

Heuristics introduced at each grade level equip students with strategies to solve increasingly complex problems. Students apply these strategies to solve real-world problems through a consistent problem-solving process.

Mathematical and Perceptual Variation

Mathematical variation presents opportunities for students to experience the same math concept through various applications. Perceptual variation showcases a mathematical concept using different representations. Variation deepens understanding as students apply the concepts in different ways.

Learning Progression

Math is learned incrementally, with one concept building on the next. More depth is added, linking new concepts to the learning that has already been mastered. Learning math this way leads to deeper conceptual understanding.

Readiness phase connects prior learning and gives students an inquiry-based opportunity to enter a task.

• Chapter Opener – the Chapter Opener stimulates curiosity and interest through a context that connects math to real life, taps into prior knowledge and encourages discussion.
• Recall – Recall helps to assess students’ readiness for the chapter so teachers can allocate appropriate resources during lessons.

Engagement Phase builds knowledge through the use of the Anchor Task, Learn, Activity! and Learn Together elements in each lesson. The engagement phase provides opportunity for productive struggle, flexible thinking, collaboration, problem solving and concept development.

• Task (Anchor Task) – relatable questions that provide opportunities for productive struggle.
• Learn – teacher guided inquiry related to the task (anchor task). It introduces the most fundamental aspect of a concept for the learning objective. Students learn through concrete experiences and visual models.
• Learn Together – consists of a series of problems that are carefully varied and progress from the simplest concept in the Learn, to more complex ones, developing and deepening students’ understanding. Learn Together is student centric.
• Activity! – requires students to solve problems collaboratively and demonstrate understanding by articulating their thinking.

Mastery Phase provides ample opportunities at the end of every lesson for independent practice and consolidation. Students develop mastery in this phase through a number of resources, including:

• Student Book
• Practice On Your Own – an independent practice at the end of a lesson that serves as formative assessment, informing teachers which resource they should assign to students for more independent practice.
• Think! – leads students to reflect on math concepts and challenges them to use different strategies to solve a non-routine problem.
• Chapter Practice – consolidates concepts and skills across a chapter through independent practice.
• Performance Task – are formative assessment tasks set in real-world context with accompanying rubrics. The questions require application of concepts and skills learned.
• STEAM Project – interdisciplinary tasks that span multiple chapters and shows the relevance and importance of mathematics. They promote critical and creative thinking.
• Solve! Heuristics – a dedicated section to teach problem-solving strategies that can be applied to different types of problems. Across the grades students will be introduced to new strategies that will help them enter and solve complex problems.
• Additional Practice – provides on-level, independent practice at the end of each lesson to consolidate skills and concepts.
• Mastery and Beyond – provides on-level, independent practice at a section level to connect multiple lessons. The Teachers Guide points out when to assign Mastery and Beyond practices.

• Across Grades Progression Charts at the beginning of each chapter shows a longitudinal progression providing an overview of prior knowledge, current grade learning objectives and future learning.
• Chapter Overview – provides embedded teacher support by providing insights into the Key Ideas of the chapter. Concrete-Pictorial-Abstract Progression provides an overview of the concrete materials used, as well as pictorial and abstract representations students will encounter in the chapter.
• Chapter Progression is an overview of what students will learn in each chapter.
• Chapter At A Glance shows the lesson objectives, focus questions, I CAN statements, new vocabulary, materials and instructional resources necessary to prepare for teaching.
• English Language Support provides strategies for teachers to guide and support students in learning and using mathematical language.
• Promoting Growth highlights common learning difficulties that students may encounter in the chapter and provides strategies to help students overcome them.
• Lesson Opener provides teaching ideas for teachers to orchestrate concrete experiences in the Task (Anchor Task).
• Lesson Development provides ideas for concrete experiences and support at different levels of mastery.
• Caution highlights common errors and provides suggestions to rectify the errors.
• Lesson Debrief wraps up the lesson by posing focus questions for students to articulate their understanding through discussion or journaling and for teachers to evaluate students’ level of understanding.
• Differentiated Instruction suggests activities for students at different levels of mastery.
• Transition Guide helps teachers to identify student learning gaps as students begin a chapter and identifies the teacher resources in the program that would help close the gap.